A little note on Shack-Hartmann wavefront sensor

A well-known Hartmann test\cite{hartmann1900} devised initially for telescope optics control was adapted for adaptive optics. The design of this sensor was based on an aperture array that had been developed by Johannes Franz Hartmann\cite{platt2001history} as a means to trace individual rays of light through the optical system of a large telescope, thereby testing the quality of the image\cite{hartmann1900}. In the late 1960s Shack and Platt\cite{shackhartmproduction} modified a Hartmann screen by replacing the apertures in an opaque screen with an array of lenslets.


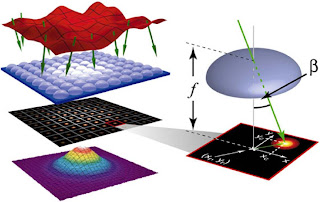
It is interesting that the construction of SH WFS is simple yet effective; in fact, first lenslet arrays were kithcen-made\cite{platt2001history}:

Platt made a mount for compression, moulding a 1-mm-thick square plate of optical grade thermal plastic (Plexiglass) between the two Cervet squares. [...] Each heating and cooling cycle in the moulding process took several hours. So, Platt decided to work all day and most of the night in his wife's kitchen. His wife's electric knife sharpener was used to trim the Plexiglass plates. Her apron and oven mittens were also used to remove the moulds from the oven. After a few weeks, the process was perfected and good quality lens arrays were being produced. For at least the next 5 years, all lens arrays used in Shack-Hartmann wavefront sensors were made by Platt in his wife's kitchen.

The principle of the Shack-Hartmann (SH) wavefront sensor (WFS) is the following. An image of the exit pupil is projected onto a lenslet array. Each lens takes a small part of the aperture (sub-aperture), and forms an image of the source. When an incoming wavefront is planar, all images are located in a regular grid defined by the lenslet array geometry. As soon as the wavefront is distorted, images become displaced from their nominal positions. Displacements of image centroids in two orthogonal directions $x,y$ are proportional to the average wavefront slopes in $x,y$ over the sub-apertures.


Centroiding
For a sampled light intensity $I_{i,j}$, positions of spots $x_{c_k}$ and $y_{c_k}$ are:

$$ x_{c_k} = \frac{\sum_{i,j} x_{i,j}I_{i,j}}{\sum_{i,j} I_{i,j}},\,\,\,\,
y_{c_k} = \frac{\sum_{i,j} y_{i,j}I_{i,j}}{\sum_{i,j} I_{i,j}},
$$

Resulting local direction angle $\beta$:
$$
\beta_x \approx (x_{c_k} - x_{r_k})\frac{L_x}{f},\,\,\,\,
\beta_y \approx (y_{c_k} - y_{r_k})\frac{L_y}{f},
$$

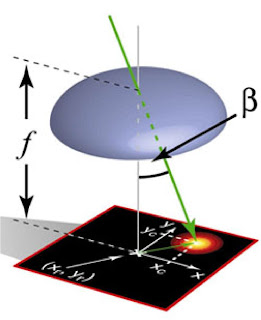where $L_x$ is a size of the photosensor's pixel and $f$ is the focus length of the lenslet.


\vspace{3ex}\textit{Advantages}: predictable results, simple and effective construction, ability to simultaneously determine $x$ and $y$ slopes.

\vspace{3ex}\textit{Disadvantages}: centroiding algorithm is computationally-intensive.

\vspace{3ex}Shack-Hartmann wavefront sensor is the most popular: its precision and accuracy can be scaled over a huge range through the choice of lenslet array and detector.



Typical WFS SH characteristics
Typical parameters for the Shack-Hartmann sensor in the year 2010 are:

• Aperture Size 5.95 mm x 4.76 mm. Max.
• Camera Resolution 1280 x 1024 Pixels Max.
• Pixel Size $4\dots5.5 \mu$m
• lenslet size 300$\mu m$
• lenslet focus distance 7 mm
• Number of Subapertures $\leq 150$

References \begin{thebibliography}{1}

\bibitem{hartmann1900}
J.~Hartmann.
\newblock {Bemerkungen {\"u}ber den Bau und die Justirung von Spektrographen}.
\newblock {\em Z. Instrumentenkd}, 20:47, 1900.

\bibitem{platt2001history}
B.C. Platt.
\newblock {History and principles of Shack-Hartmann wavefront sensing}.
\newblock {\em Journal of Refractive Surgery}, 17(5):573--577, 2001.

\bibitem{shackhartmproduction}
R.V. Shack and B.C. Platt.
\newblock {Production and use of a lenticular Hartmann screen}.
\newblock {\em J. Opt. Soc. Am}, 61(5):656, 1971.

\end{thebibliography}