When the Poisson distribution can be approximated as Gaussian?

In many articles that deal with noise, and especially in photosensors, there is a usual mantra assume the noise as a Gaussian distributed. Such mantra is said even to processes that are Poissonian by the very nature (photon shot noise). The question: ``how many arrivals can justify the Gaussian approximation?'' is usually answered in vague term ``many''. Well, how many!?

Good question. Some authors say\cite{mendenhallstatistics} that more then 1000 arrival events can be considered as Gaussian. And it seems to be reasonable, as shown [the picture from here]:

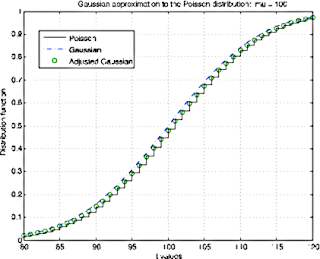
The approximation of Poisson distribution by Gaussian is performed usually to simplify the calculations. However, the approximation is not simple. In\cite{poisson2gaussapprox} was shown that the usual approximation of a Poisson density function with mean $m$ by a Gaussian density function with mean $\mu$ and variance $\sigma$ both equal to $m$ is in general not satisfactory for error-rate calculations. A short letter to IEEE Transactions describes a modified Gaussian approximation to a Poisson distribution which, unlike the usual Gaussian approximation, gives good agreement on the tails of the distribution.

We can express Poisson distribution $p(n, m)$ in terms of Gaussian $g(n,m)$ as follows\cite{poisson2gaussapprox}:

$$p(n,m) = \left(\frac{1}{\sqrt{2\pi m}}\right)^{\frac{1}{1+\alpha}} \exp\left[ -\frac{\beta}{1+\alpha} - \frac{(n-m)^2}{2m(1+\alpha)} \right] $$

Thus a Gaussian that better approximates Poisson $p(n, m)$ on the tails has the same mean as $p(n, m)$ but has variance $m(1 + \alpha)$.

The parameters $\alpha$ and $\beta$ can be expressed via $\mu$ as:

$\alpha = (0.080\mu^2 - 0.230\mu + 1.260)^{-2}$

and

$\beta = (0.0667\mu^2 -0.3933\mu + 0.810)^{-1}$

References:

\begin{thebibliography}{1}

\bibitem{mendenhallstatistics}
W.~Mendenhall and T.~Sincich.
\newblock {Statistics for Engineering and the Sciences}.
\newblock 2006.

\bibitem{poisson2gaussapprox}
W.M. Hubbard.
\newblock The approximation of a poisson distribution by a gaussian
distribution.
\newblock {\em Proceedings of the IEEE}, 58(9):1374 -- 1375, 1970.

\end{thebibliography}